Duncan's paradox
Duncan's paradox is a thermodynamic thought experiment proposed by T. Duncan in 2000 [1] that has connections to the second law of thermodynamics and catalysis.
Duncan's paradox was devised to demonstrate the incompatibility of these purported pressure gradients with the second law of thermodynamics. Specifically, such pressure gradients would allow construction of perpetual motion machines of the second type; therefore, because such devices are presumed thermodynamically impossible, the enabling pressure gradients must also be impossible.
Pressure and temperature paradoxes[edit]
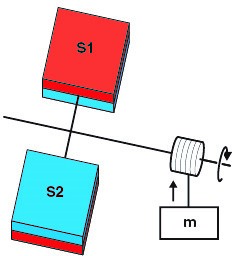
Duncan's paradox can be envisioned either in terms of pressure or temperature differences. Consider a dimeric gas (A2) that is susceptible to endothermic dissociation or exothermic recombination (A2 ↔ 2A). The gas is housed between two surfaces (S1 and S2), whose chemical reactivities are distinct with respect to the gas. Specifically, let S1 preferentially dissociate dimer A2 and desorb monomer A, while S2 preferentially recombines monomers A and desorbs dimer A2.

For the pressure paradox, let S1 and S2 cover the apposing faces of a radiometer, which is housed inside a sealed blackbody cavity, itself lined with S2 (Fig. 1). Let the surface area of the vanes be much less than that of the cavity walls so that the cavity gas concentrations are effectively set by the gas-surface reactions on the walls. Because S1 preferentially desorbs monomers compared with S2, and because two monomers are desorbed for every dimer, it can be shown, for a broad range of parameters, that there will be a greater gas pressure over the S1 than over the S2 vane faces. This steady-state pressure differential turns the radiometer and can be harnessed to perform steady-state work; in this case (Fig. 1), a weight is lifted against gravity.
Appealing to the first law of thermodynamics, it can be shown that this steady-state work must come ultimately from heat from the heat bath; however, this contradicts the Kelvin-Planck form of the second law, which forbids the conversion of heat solely into work in a thermodynamic cycle such as this. Thus, if the second law is inviolable, such pressure differences must be thermodynamically impossible.
Duncan's temperature paradox follows similarly from this. Here, surface desorbed A2 and A set up a convective cycle between S1 and S2 (Fig. 2). S1 provides an excess of A to S2, while S2 provides an excess of A2 to S1. Because the reaction A2 → 2A is endothermic—and its reverse exothermic—it follows that S1 cools down and S2 heats up. The permanent temperature difference thus established between S1 and S2 can be harnessed via a heat engine (e.g., a thermoelectric device) to perform work, again as before, at the ultimate expense of the surrounding heat bath and in violation of the Kelvin-Planck form of the second law. As proposed by Duncan for his pressure paradox, this temperature paradox also would be resolved by forbidding the existence of differential gas-surface products between different surface types.
See also[edit]
References[edit]
- ↑ Duncan, T.L. (2000). "Comment on "Dynamically maintained steady-state pressure gradients"". Phys. Rev. E. 61: 4661. Bibcode:2000PhRvE..61.4661D. doi:10.1103/PhysRevE.61.4661.CS1 maint: Uses authors parameter (link)
This article "Duncan's paradox" is from Wikipedia. The list of its authors can be seen in its historical and/or the page Edithistory:Duncan's paradox. Articles copied from Draft Namespace on Wikipedia could be seen on the Draft Namespace of Wikipedia and not main one.
