Ecological Structural Instability
Script error: No such module "Draft topics".
Script error: No such module "AfC topic".
Ecological Structural Instability refers to the sensitivity of ecological communities to direct or indirect environmental pressures which are so high that they result in species extinctions.[1]. At this point, invasion of a single new species can force the extinction of another. Species of a low abundance with small biomass are the most likely to go extinct at the point of Ecological Structural Instability[2]. If one species replaces the role of another species upon extinction, then this will have little effect on ecosystem function[1]
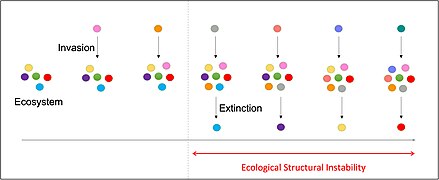
This links to the mathematical concept of structural instability, defined in dynamical systems theory as a situation where a small change in a system can change its behaviour qualitatively.[3].
Effects of invaders[4]
Invaders can impact communities directly through:
• Direct interspecific competition between species (e.g. for shelter)
Or indirectly through:
• Interspecific competition of predators for shared prey.
• Introduction of a new predator leading to trophic cascades
Near the instability limit, indirect interactions involving multiple species are important, accounting for ~50% of community alterations [5][6]. The full effects of this would be seen over a long period of time, so short-term responses are a poor measure of the full impact[7].
Mathematical Models
Mathematical analysis can provide more in depth understanding of ecological structural instability, especially because the effects of indirect interactions can be counter-intuitive, and can be used to make quantitative predictions.
Lotka-Volterra competition models with random interactions aid the study of instability in species-rich communities and are supported by empirical data [8]. Simulation predictions are often robust to variations in model structure, except in some cases [9][10]
Mathematical analysis shows that around half of invasion attempts succeed, matching observations [11].
References[edit]
- ↑ 1.0 1.1 Rossberg, Axel; Caskenette, Amanda; Bersier, Louis-Félix (5 December 2017). Adaptive Food Webs. Cambridge: Cambridge University Press. pp. 372–383. doi:10.1017/9781316871867.024. Retrieved 1 December 2023. Search this book on

- ↑ "Biodiversity and Ecosystem Stability | Learn Science at Scitable". www.nature.com. Retrieved 2024-04-16.
- ↑ Pugh, Charles C.; Robinson, Clark (June 1983). "The C1 Closing Lemma, including Hamiltonians". Ergodic Theory and Dynamical Systems. 3 (2): 261–313. doi:10.1017/S0143385700001978. ISSN 1469-4417.
- ↑ Northfield, Tobin D.; Laurance, Susan G. W.; Mayfield, Margaret M.; Paini, Dean R.; Snyder, William E.; Stouffer, Daniel B.; Wright, Jeffrey T.; Lach, Lori (2018-01-31). "Native turncoats and indirect facilitation of species invasions". Proceedings of the Royal Society B: Biological Sciences. 285 (1871): 20171936. doi:10.1098/rspb.2017.1936. ISSN 0962-8452. PMC 5805925. PMID 29367390.
- ↑ Menge, Bruce (1995). "Indirect Effects in Marine Rocky Intertidal Interaction Webs: Patterns and Importance". Ecological Monographs. 65 (1): 21–74. Bibcode:1995EcoM...65...21M. doi:10.2307/2937158. JSTOR 2937158. Retrieved 1 December 2023.
- ↑ Rossberg, Axel (3 June 2013). Food Webs and Biodiversity: Foundations, Models and Data. John Wiley & Sons, Ltd. doi:10.1002/9781118502181. ISBN 9781118502181. Retrieved 1 December 2023. Search this book on

- ↑ Zelnik, Yuval R.; Galiana, Nuria; Barbier, Matthieu; Loreau, Michel; Galbraith, Eric; Arnoldi, Jean-François (2024). "How collectively integrated are ecological communities?". Ecology Letters. 27 (1): e14358. Bibcode:2024EcolL..27E4358Z. doi:10.1111/ele.14358. ISSN 1461-023X. PMID 38288867 Check
|pmid=value (help). - ↑ Barbier, Matthieu; de Mazancourt, Claire; Loreau, Michel; Bunin, Guy (2021-01-14). "Fingerprints of High-Dimensional Coexistence in Complex Ecosystems". Physical Review X. 11 (1): 011009. Bibcode:2021PhRvX..11a1009B. doi:10.1103/PhysRevX.11.011009.
- ↑ Barbier, Matthieu; Arnoldi, Jean-François; Bunin, Guy; Loreau, Michel (2018-02-27). "Generic assembly patterns in complex ecological communities". Proceedings of the National Academy of Sciences. 115 (9): 2156–2161. Bibcode:2018PNAS..115.2156B. doi:10.1073/pnas.1710352115. ISSN 0027-8424. PMC 5834670. PMID 29440487.
- ↑ Pettersson, Susanne; Savage, Van M.; Jacobi, Martin Nilsson (2020-12-03). "Stability of ecosystems enhanced by species-interaction constraints". Physical Review E. 102 (6): 062405. Bibcode:2020PhRvE.102f2405P. doi:10.1103/PhysRevE.102.062405. PMID 33465982 Check
|pmid=value (help). - ↑ Cockrell, Cillian; O'Sullivan, Jacob D.; Terry, J. Christopher D.; Nwankwo, Emmanuel C.; Trachenko, Kostya; Rossberg, Axel G. (2024-01-25). "Self-organization of ecosystems to exclude half of all potential invaders". Physical Review Research. 6 (1): 013093. Bibcode:2024PhRvR...6a3093C. doi:10.1103/PhysRevResearch.6.013093.
This article "Ecological Structural Instability" is from Wikipedia. The list of its authors can be seen in its historical and/or the page Edithistory:Ecological Structural Instability. Articles copied from Draft Namespace on Wikipedia could be seen on the Draft Namespace of Wikipedia and not main one.
