Car speed and energy consumption

A car splashes through a minor flood on Henbury Road, Bristol, 2012. Here the car loses energy due to heat dissipation, air and water resistance, and rolling resistance of the tyres on the road surface which should all be accounted for in a calculation of the required car energy.

The energy consumption of a car covering a given distance for locomotion is found to depend on the speed of the vehicle. The faster a car drives, the more energy is required for propulsion. At speeds below 40 km/h, energy for a given distance is roughly constant with speed; at higher speed the energy consumption of a car per unit distance increases proportional to the square of the speed. This is caused by the energy loss due to air drag which at high car speeds exceeds the energy losses due to both heat production and car tyre rolling resistance.

Due to the quadratic growth of air drag with car speed, driving twice as fast costs approximately four times as much fuel per unit of distance at high speeds. The required power increases with the cube of the speed, so driving twice as fast costs theoretically eight times as much fuel per unit of time.

Mechanisms for energy loss at moderate speed

An average passenger car dissipates fuel energy through various mechanisms. For a speed of 60 km/h, Holmberg et al. (2012) found the following breakup by percentages from the literature:

1. 33% (range, 30–37%): heat loss in exhaust gases
2. 29% (25–33%): heat loss in the engine, the radiator, and the car’s heating system
3. 38% (33–40%): air drag and friction losses, subdivided into:
   1. 5% (3–12%): air drag
   2. 33%: rolling resistance and friction in the car.

MacKay's simulation shown in Graph 1 largely concurs while assuming slightly different car parameters, while not considering the heat losses. At low to moderate speeds the energy loss due to air drag is dwarfed by the heat losses. However, at higher speeds energy loss by air drag comes to dominate the other mechanisms.

Energy loss by acceleration, air drag and rolling resistance at various speeds

Graph 1. Plot of the energy a car consumes versus its speed. Air resistance is the main cause of the car fuel consumption (expended energy per distance) when driving at high steady speeds. Assumptions: the car's engine uses energy with an efficiency of 0.25, whatever the speed; $c_{d}\cdot A_{car}$ = 1 m², and $m_{car}$ = 1000 kg, and $C_{rr}$ = 0.01. From David J.C. MacKay: Sustainable energy - without the hot air (2009), page 259, Figure 321.

Graph 2. At low speeds, the fuel consumption of Prius and BMW 318ti cars do not vary proportional to the square of their speeds. However at higher speeds, the Prius data approximately do.
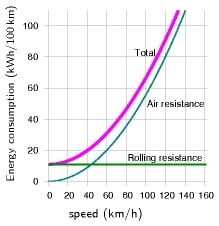
Horizontal green line: rolling resistance. Green curve: air resistance. Pink curve: Total, rolling and air resistance summed. From David J.C. MacKay: Sustainable energy - without the hot air (2009), page 260, Figure 256.

The energy $E_{\text{motor}}$ for propulsion supplied by a car's engine is spent on:

1. accelerating the car (abbreviated to ac),
2. overcoming the air resistance (aerodynamic drag, dr), and
3. overcoming the rolling resistance (rr).

so that

$E_{\text{motor}}=E_{\text{ac}}+E_{\text{dr}}+E_{\text{rr}}$

The energy effects of wind, ascent and descent are not considered here, nor the repeated braking and accelerating when driving in a city.

Acceleration

The kinetic energy $E_{\text{kin}}$ of a moving car with mass $m_{car}$ and speed $v_{car}$ can be calculated theoretically as

$E_{\text{kin}}={\tfrac {1}{2}}m_{car}\cdot v_{car}^{2}$

so for a car with a mass of 1,000 kg at a speed of 120 km/h (33.3 m/s)

$E_{\text{kin}}=0{.}5\times 1000\times 33{.}3^{2}=5{.}56\times 10^{5}\,{\text{J}}=0{.}15\,{\text{kWh}}$

(One kilowatt hour (kWh) equals 3.6 million joules (J).)

The required kinetic energy is supplied by the motor when the car accelerates starting from rest. It is lost when the driver brakes (unless the available kinetic energy is stored in, for example, a flywheel). Part of this energy can also be recovered from electric cars via recuperative braking and can be stored in a accumulator.

In practice, the real energy consumption of a car depends on many additional factors, including the traffic situation and personal driving style. In order to be able to make comparisons, standardized consumption tests have been devised, driving cycles, such as the New European Driving Cycle (NEDC) and the newer Worldwide harmonized Light vehicles Test Procedures (WLTP).

Without any resistance or friction, by Newton's First Law a car with speed $v$ would continue at this speed forever. However, air drag and rolling resistance cause energy losses which must be overcome to drive at a constant speed.

Air drag

Depending on its surface area $A_{car}$, streamlining and speed $v$, a car moves a lot of air, which causes energy loss by the aerodynamic drag force. We can imagine the moving air as a tube (trunk) of air behind the car, with a volume of the cross-sectional area of that tube times the length, which equals the distance traveled by the car. This length is then equal to the car speed times the elapsed time. The effective cross-section of the air tube is less than the front surface of the car $A_{car}$, for example due to streamlining. This can be taken in account by introducing a drag coefficient $c_{drag}$ for the air resistance of about 1/3.
Suppose the surface area of the wind shield of a car is

$A_{car}=2m\times 1.5m=3m^{2}$

then the effective volume $V_{eff-air}$ of that tube of swirling air behind the car, after a period of driving $t$ at speed $v$ equals

$V_{eff-air}=A_{car}\cdot tubelength=A_{car}\cdot c_{drag}\cdot v_{car}\cdot t$

with $v_{car}\cdot t$ = $d$ the distance traveled.
The mass of the displaced air over a journey of $d$ meters is equal to the volume of the tube times the specific mass $\rho _{air}$ of air, so

$m_{air}=V_{eff-air}\cdot \rho _{air}=3\times 1/3\cdot d\cdot \rho _{air}$.

The energy loss due to air resistance is equal to the kinetic energy that the car imparts to the air.
Using the value of $1.3kgm^{-3}$ for the density of air $\rho _{air}$ results in

$E_{drag}={\tfrac {1}{2}}m_{air}\cdot v_{car}^{2}={\tfrac {1}{2}}\rho _{air}\cdot A_{car}\cdot d\cdot c_{drag}\cdot v_{car}^{2}\cong 0.65\cdot v_{car}^{2}\cdot d$

Rolling resistance

The mathematical formula for the force due to the rolling resistance of the car tyres is:

$F_{rr}=C_{rr}\cdot m\cdot g$

with

$C_{rr}$ the roll coefficient which is around 0.01.

$m$ the mass of the car, and

$g$ the gravitational acceleration.

The corresponding energy loss caused by the rolling resistance is (the work done by the rolling resistance)

$E_{rr}=F_{rr}\cdot d=constant\cdot d$,

with $d$ the distance covered, so the energy spent on overcoming the rolling resistance per unit distance is a constant.

Total energy consumption to overcome friction

Adding up the energy consumption required to overcome both air drag and tyre rolling resistance, we have

$E_{\text{total car over distance d}}=E_{\text{air resistance}}+E_{\text{rolling resistance}}$

$E_{drag}\cong 0.65\cdot v_{car}^{2}\cdot d+constant\cdot d$

From the graphs it is seen that per unit distance the drag resistance dominates over the rolling resistance at higher car speeds.

$E_{\text{tot}}\cong 0{.}7\cdot v^{2}\cdot d$

The energy consumption over a distance and the air pollution are proportional to the speed squared

The energy consumption of a car at higher speeds is seen to be proportional to its speed $v$ squared, and of course also to the distance $d$ traveled. So if the air resistance on the car dominates – and acceleration/braking and rolling resistance are of less significance for energy consumption, such as on the highway – the energy consumption is proportional to the square of the car speed. If air pollution increases in line with petrol consumption, this means that traveling at 120 km/h over the same distance pollutes 15% less than at 130 km/hour, because (120/130)² = 0.85 = 85% and 100% - 85% = 15%.

Because a car mainly produces heat and only uses 25% of the energy from the fuel for movement (an electric car uses about 90% for movement), the petrol must provide much more energy than the car uses for movement:

$E_{\text{supplied by gasoline}}=4\times E_{tot}\cong 2{,}8\cdot v^{2}\cdot d$

Car power is proportional to the cube of the speed

Graph 3. Car power scales with the third power of the top speed for different car brands (Ferrari, Porsche, Opel Kadett, and Panda). Logarithmic scales. Tennekes, H.: Simple Science of Flight, MIT Press 2009. Used by David J.C. MacKay: Sustainable Energy - without the hot air, 2009.

Precise details of a car's energy consumption depend on the construction etc. of a car, but in general this quadratic relationship with speed is correct for speeds above 60 km/h, but refer to the accompanying figure. This can also be seen in the measured relationship between the power (energy per time, so energy times speed divided by distance) $P_{car}$ of cars and the cube of their top speeds $v_{\text{top}}$

$P_{top,car}=E_{top,car}/t=E_{top,car}/(d/v_{\text{top}})=E_{top,car}\cdot v_{\text{top}}/d\cong v_{\text{top}}^{3}$, so

$P_{top,car}\cong v_{\text{top}}^{3}$

Energy is proportional to the square of the speed and must be multiplied again by the speed to obtain the expended power. So the car power scales with the third power of the car speed. The consumption of energy per second increases eightfold (2³) if you drive twice faster.

Friction dominates at low speed

At 60km/h, the global average speed, energy loss due to air drag in fossil fuel cars is approximately 5% of the total energy loss. Friction (33%), exhaust (29%), and cooling the engine (33%) account for the rest. Electric vehicles are estimated to have about half as much loss due to friction.

See also

• Energy efficiency in transport
• Energy-efficient driving
• Fuel economy in automobiles
• Kinetic energy

Further reading

• Benson, Harris (1996). University Physics Revised edition. New York: John Wiley & Sons. pp. 144–145. ISBN 9780471006893. OCLC 1403779613. Search this book on
• Fundamentals of Physics: Halliday, David; Resnick, Robert; Walker, Jearl (1993). Fundamentals of physics. Fourth edition. Extended, with modern physics (4th ed.). New York, Chichester, Brisbane, Toronto, Singapore: John Wiley & Sons. ISBN 0471600121. OCLC 31514817. Search this book on
• On the related topic of friction:
  • Holmberg, Kenneth; Andersson, Peter; Erdemir, Ali (March 2012). "Global energy consumption due to friction in passenger cars". Tribology International. 47: 221–234. doi:10.1016/j.triboint.2011.11.022. Retrieved 21 November 2023.
  • Holmberg, Kenneth; Andersson, Peter; Siilasto, Roope; Erdemir, Ali (May 2013). "Global energy consumption due to friction in passenger cars, transportation and industry STLE Annual Meeting, Detroit, USA, 5-9.5.2013" (PDF). Retrieved 21 November 2023.
  • Holmberg, Kenneth; Erdemir, Ali (6 September 2017). "Influence of tribology on global energy consumption, costs and emissions". Friction. Springer Link. 5 (3): 263–284. doi:10.1007/s40544-017-0183-5. Retrieved 21 November 2023.

External links

• MacKay, David (29 August 2015). "Sustainable Energy – without the hot air". withouthotair.com. Retrieved 22 October 2023. Previous book publication 2009. PDF free download.
• Physics Classroom (2023). "Kinetic Energy". The Physics Classroom. Retrieved 2023-10-22.

This article "Car speed and energy consumption" is from Wikipedia. The list of its authors can be seen in its historical and/or the page Edithistory:Car speed and energy consumption. Articles copied from Draft Namespace on Wikipedia could be seen on the Draft Namespace of Wikipedia and not main one.

📰 Article(s) of the same category(ies)

• Consolidated power generation
• Energy efficient clay brick project
• EcoCute
• ETC Group (energy efficiency consultant)
• Bill Lishman's underground house
• Energy Elephant (company)
• Jerome Johnson (entrepreneur)

---